- 6.1 Implicit Vs Explicitap Calculus Calculator
- 6.1 Implicit Vs Explicitap Calculus Solver
- 6.1 Implicit Vs Explicitap Calculus 2nd Edition
- 6.1 Implicit Vs Explicitap Calculus Algebra
So, what have I done? `(int1^2 x^5 dx = x^6/61^2=2^6/6-1^6/6)` I've found the integral, and I've substituted 2 into this position. And 1 into this position. And I'm going to subtract the difference. And I'm just going to use Scientific Notebook now to give me that, as a decimal. And it turns out to be 10.5.
| There are two ways to define functions, implicitly andexplicitly. Most of the equations we have dealt with havebeen explicit equations, such asy = 2x-3, so that we can writey = f(x) wheref(x) = 2x-3. But theequation 2x-y = 3 describes the samefunction. This second equation is an implicit definition ofy as a function of x. As there is no realdistinction between the appearance of x or y inthe second form, this equation is also an implicit definition ofx as a function of y |
- 6.3 Explicit Vs Implicit Differentiation Notes No tes Key. Homework Hw Key. Powered by Create your own unique website with customizable templates.
- What is Implicit Differentiation? 🎥Watch: AP Calculus AB/BC - Implicit Derivatives. Back in pre-calculus, you likely learned or talked about how there are two different types of equations: explicit equations and implicit equations. An explicit equation is written such that y is isolated on one side. For example, y=2x+3 is an explicit equation.
Not all implicit equations can be restated explicitly in a singleequation. For example, the implicit equationx2+y2 = 9 needstwo explicit equations,
which are the top and bottom halves of acricle respectively, to define the functional relation completely.
| And xy =sin(y)+x2y2(the magenta curves in the figure at the left) cannot be solved foreither y as an explicit function of x orx as an explicit function of y. This implicitfunction is considered in Example 2. |
Perhaps surprisingly, we can take the derivative of implicit functionsjust as we take the derivative of explicit functions. We simply takethe derivative of each side of the equation, remembering to treat thedependent variable as a function of the independent variable, apply therules of differentiation, and solve for the derivative. Returning toour original example:
This is of course what we get from differentiating the explicit form,y = 2x-3, with respect to x.
This simple example may not be very enlightening. Consider thesecond example, the equation that describes a circle of radius 3,centered at the origin. Taking the derivative of both sides withrespect to x, using the power rule for the derivative ofy,
It can be seen from the figures that for either part of the circle,the slope of the tangent line has the opposite sign of the ratiox/y, and that the magnitude of the slope becomeslarger as the tangent point nears the x-axis.
Deeper sleepclout games free. (For this case, finding dy/dx as an explicit function ofx requires use of the power rule for fractional powers,usually considered later. This example may be thought of as a taste ofthings to come.)
Some examples:

6.1 Implicit Vs Explicitap Calculus Calculator
Note that this expression can be solved to give x as anexplicit function of y by solving a cubic equation, andfinding y as an explicit function of x wouldinvolve soving a quartic equation, neither of which is in ourplan.
Using the chain rule and treating y asan implicit function of x,
As in most cases that require implicit differentiation, the result inin terms of both x and y. In general, if givingthe result in terms of x alone were possible, the originalexpresson could be solved for y as an explicit function ofx, and implicit differentiation, while still correct, wouldnot be necessary.
In this case, the chain rule and product rule are both used toadvantage:
The use of inverse trigonometric functions allow this to be solve fory as an explicit function of x and graphed, asshown. However, this function serves as a good example of implicitdifferentiation:
6.1 Implicit Vs Explicitap Calculus Solver
In implicit differentiation, and in differential calculus in general,the chain rule is the most important thing to remember!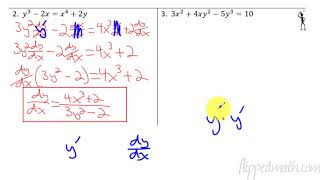

Exercises:
6.1 Implicit Vs Explicitap Calculus 2nd Edition
- Differentiate the following equations explicity, findingy as a function of x. Solve fory´=dy/dx.
6.1 Implicit Vs Explicitap Calculus Algebra
