- 1.7 Intermediate Value Theorem Ap Calculus Calculator
- 1.7 Intermediate Value Theorem Ap Calculus Frq
- 1.7 Intermediate Value Theoremap Calculus Solver
- Mean Value Theorem
- Intermediate Value Theorem Examples
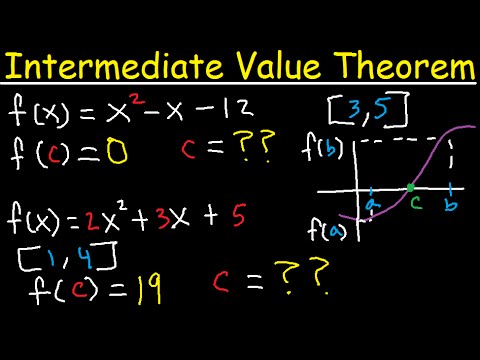
Intermediate Value Theorem to support the conclusion and did not earn the second point. In part (b) the student does not calculate the difference quotient and was not eligible for either point. In part (c) the student earned both points by correctly applying the Fundamental Theorem of Calculus and the chain rule and by correctly evaluating. Since it verifies the intermediate value theorem, the function exists at all values in the interval 1,5. Solution of exercise 4 Using Bolzano's theorem, show that the equation: x³+ x − 5 = 0, has at least one solution for x = a such that 1. A More Formal Definition. The textbook definition of the intermediate value theorem states that: If f is continuous over a,b, and y 0 is a real number between f (a) and f (b), then there is a number, c, in the interval a,b such that f (c) = y 0. In other words, if you have a continuous function and have a particular “y” value, there must be an “x” value to match it. 1.7 Intermediate Value Theorem. Powered by Create your own unique website with customizable templates. Stack Exchange network consists of 176 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers.
Find limits using the Squeeze Theorem.
Squeeze Theorem
In this section we find limits using the Squeeze Theorem.
1.7 Intermediate Value Theorem Ap Calculus Calculator
Since and we can use the Squeeze Theorem to conclude that as well.
First, we need to find bounds. Since for all , for all except . Next, we need to findthe limits of those bounds: Since these answers are the same, the Squeeze Theorem allows us to conclude that
The Squeeze Theorem can also be used if .
First, we need to find bounds. Since for all , for all . Next, we need to find the limitsof those bounds: Since these answers are the same, the Squeeze Theorem allows us toconclude that
The area of the small triangle is The area of the sector is The area of the largetriangle is
We can use the areas of these figures to create a compound inequality like the onefound in the Squeeze theorem. Since the area of the small triangle is less than thearea of the sector which is less than the area of the large triangle, we have:
Multiply through by 2:
Divide through by . Note that if is a small positive angle, then so the direction ofthe inequality symbols remains unchanged:
Next we take reciprocals (this will change the direction of the inequalitiysymbols):
which is equivalent to
1.7 Intermediate Value Theorem Ap Calculus Frq
We now compute the limits of the upper and lower bounds:
1.7 Intermediate Value Theoremap Calculus Solver
Since the above limits are equal, by the Squeeze Theorem

Mean Value Theorem
To compute the left-hand limit, we recall that for any angle . Therefore, whichimplies that the left-hand limit and the right-hand limit are equal:
Intermediate Value Theorem Examples
Thus the two-sided limit exists and
